Here are some of the more specific research topics I am interested in. Follow the links to find out more!
-
Projections of fractals -
Marstrand type theorems and applications
-
The distance set problem -
a widely studied open problem in GMT
-
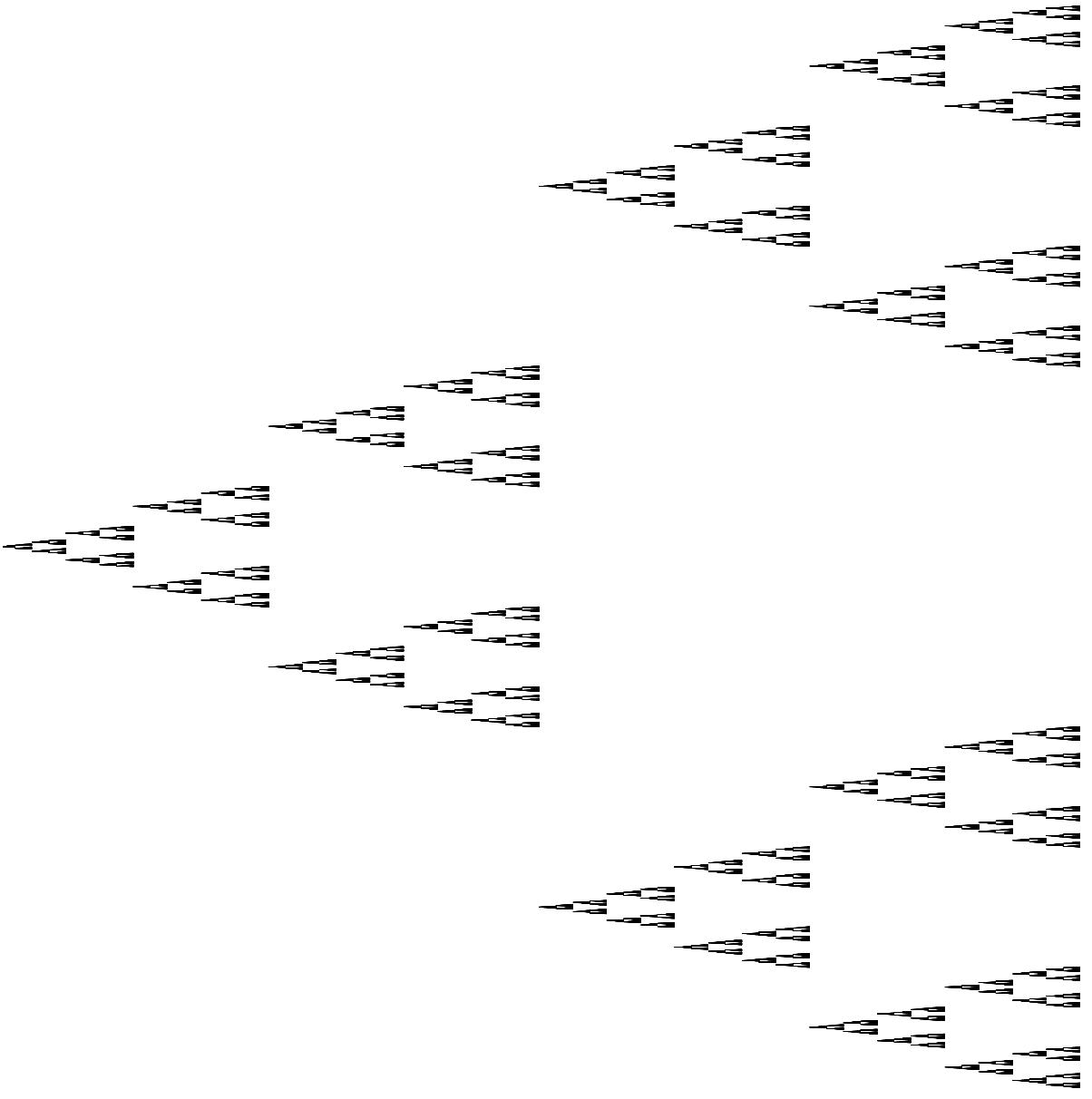
Self-affine sets and measures -
self-affine carpets, multifractals
-
Fourier analysis -
harmonic analysis, Fourier analytic techniques in fractal geometry, Fourier dimension
-
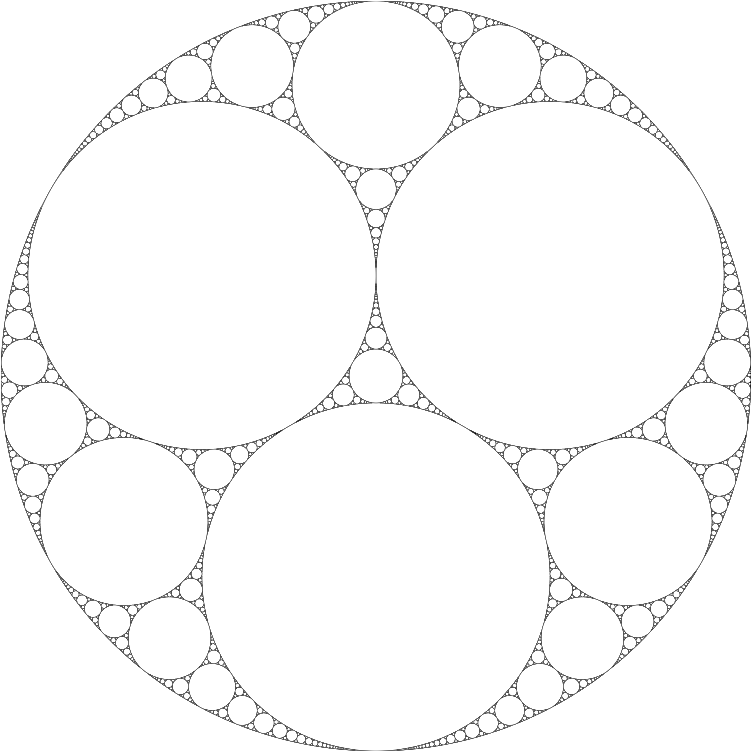
Conformal dynamics -
Kleinian groups, Julia sets, conformal IFSs
-
Kakeya type problems -
Kakeya sets, (d,k)-sets, Furstenberg sets
-
Dimension interpolation -
Assouad spectrum, intermediate dimensions, Fourier spectrum
-
Arithmetic combinatorics -
finite fields, sumsets, (approximate) arithmetic structure in fractals and sets of integers
-
Inhomogeneous IFSs -
beautiful variant of standard IFS due to Barnsley and Demko
-
Self-similar sets and measures -
self-similar sets with overlaps
 |
 |
 |
Left: a self-affine carpet of the type introduced by Bedford and McMullen. Its lower, Hausdorff, box, and Assouad dimension are all distinct. Centre: the Apollonian circle packing. It can be realised as the limit set of a Kleinian group. Right: the graph of the popcorn function. Together with Haipeng Chen and Han Yu I proved that it has box dimension 4/3.